Latitude and Longitude – Geographic Coordinates

Geographic coordinate system

Geographic coordinate system is a common spherical coordinate reference system for specifying location of features on the curved surface of the earth. A network of intersecting lines consisting of north-south lines of longitude and east-west lines of latitude creates an imaginary mesh around the globe. Such a network (graticule) allows for the description of the position of any point on the surface of the earth.

Geographic coordinates of a point is a set of two numbers, namely latitude and longitude (often abbreviated as lat long) that specify the location of that point. The two coordinates are angular measurements. Latitude provides an angular measure of the distance of the point north or south of the equator, while longitude refers to the east-west angular measure relative to the prime meridian.

Latitude – Line of Latitude

Latitude and longitude on ellipsoidal earth
(geodetic latitude)


Latitude and longitude on spherical earth
(geocentric latitude)
Graticule spacing of 10 degrees

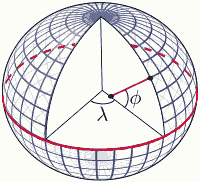
Latitude (geodetic latitude) of a point is the angle (φ) between the plane of the equator and a line passing through the point perpendicular to the surface of the ellipsoidal earth. The line drawn from the point on earth's surface does not pass through the center of the earth except for points on the poles and the equator. When referring to latitude usually the geodetic latitude is meant.

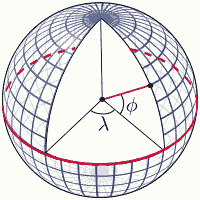
Geocentric latitude of a point on the surface of the earth is the angle between the plane of the equator and the line connecting the point to the center of the spherical earth.

Latitude can be thought of as the angular distance of a point (on the surface of earth) north or south of the equator. Since the plane of equator is the reference plane for measuring latitudes, equator is at 0° latitude. Equatorial plane divides the earth into two equal hemispheres. Latitudes are designated with N (north) for locations north of the equator and with S (south) for locations south of the equator. Latitude angles may also be assigned positive values for northern hemisphere and negative values for southern hemisphere. North Pole is at 90°N, and South Pole's latitude is 90°S or -90°.

A line (arc) on the globe or on a map running east–west and connecting all points with the same latitudinal angle is called a line of latitude, parallel or a circle of latitude. Lines of latitude form circles around the earth parallel to the equator and each other. Equator is a great circle with a circumference equal to the earth's and centered on the center of the earth. Parallels run equally spaced from 0° at the equator to 90° at the poles with successively smaller diameters approaching the poles. Other than the equator, parallels have a smaller circumference than the earth, and their center does not coincide with that of the earth, and thus they are small circles. Parallels at the North and South poles are effectively points rather than circles. The distances between each degree of latitude are more or less constant and are close to 111 km, 69 statute miles or 60 nautical miles.

Note that latitude is the angle; thus a point at 35° north latitude refers to the angle of 35° between the plane of equator and the line connecting this point to the center of earth. Parallel or line of latitude is the line; the line connecting all points at 35° latitude is called the 35th parallel. Both refer to the distance in degrees north of the equator.

Latitude and Geographic Zones

The degrees of latitude north or south of the equator and the season of the year affect the amount of solar energy received in different regions of the earth. As a result, different geographical zones can be categorized based on their characteristic climate and environment. Such latitudinal geographic zones include equatorial and tropical, subtropical, midlatitude, subarctic or subantarctic, and arctic or antarctic.

Lines of latitude or parallels of importance include: Tropic of Cancer at 23.5°N parallel, Tropic of Capricorn at 23.5°S parallel, equator at 0°, Arctic Circle at 66.5°N parallel, and Antarctic Circle at 66.5°S parallel.

Longitude – Line of Longitude

The longitude of a point on the surface of the earth is the angular distance (λ) east or west from a reference line called prime meridian that runs from North Pole to South Pole. Being a reference line or origin for measuring angles, the prime meridian has a longitude of 0°.

Historically there have been several reference lines for measuring longitude. The present prime meridian was defined by an international agreement in 1884 to be an imaginary line connecting North Pole to South Pole and passing through Greenwich, England. Prime Meridian is also referred to as Greenwich Meridian.

A line (arc) on the globe or on a map running north–south connecting North Pole to South Pole and through all points with the same longitudinal angle is called a line of longitude or a meridian. Each meridian forms half of a great circle, assuming a spherical earth. Meridians intersect lines of latitude (parallels) at right angles. The spherical earth is divided into 360 degrees east–west. Lines of longitude range from 0° to 180° to the east and from 0° to -180° to the west of the prime meridian. 180° east or west longitude is the meridian opposite the prime meridian and coincides with the International Date Line (IDL has some deviations from the straight meridian line). The plane intersecting the earth at prime meridian and 180° meridian divides the earth into western and eastern hemispheres. Longitudes are designated with E (east) for locations east of the prime meridian and with W (west) for locations west of the prime meridian. Longitude angles may also be assigned positive values for the eastern hemisphere and negative values for western hemisphere.

Similar to latitude, longitude is the angle; a point being at 122° longitude refers to the horizontal angle of 122° between the line connecting the point to the center of the earth and the prime meridian's plane. Meridian or line of longitude is the line; thus the line connecting all points at -122° longitude is the 122°W meridian. Both refer to the distance in degrees from the prime meridian.

On a spherical reference surface of the earth such as a globe, lines of longitude are not parallel (as opposed to lines of latitude), rather they converge toward the poles. The ground distance of a degree of longitude is at its greatest (about 111km or 69 miles) at the equator and decreases to 0 at the poles. At 40° latitude, one degree of longitude is about 85.4km, and at 60° latitude, it spans a distance of around 55.8km.

Lines of latitude and longitude form an imaginary grid that covers the globe. Such a network of parallels and meridians is known as the graticule. The combination of latitude and longitude angles comprises the geographic coordinates of a point on the surface of the earth, and allows for the specification of its position. When specifying the coordinates of a point latitude is written before longitude.

Latitude longitude coordinates – formats and conversion

In order to be able to describe the position of a point on the surface of the earth with acceptable accuracy, smaller sections of the angular measurement compared to degrees should be used. For example, as mentioned a degree of latitude is about 111km, such a distance does not allow for exact pinpointing of the position of a point between 35th and 36th parallel above the equator. As a result degrees of latitude and longitude are further subdivided into smaller sections. Each degree ( ° ) is divided into 60 minutes of arc ( ′ ), and each minute is divided into 60 seconds of arc ( ″ ). 1° = 60′ = 3600″; and 1′ = 60″. A latitude or longitude coordinate may be written in one of three formats.

Degrees Minutes Seconds (DMS) – ddd° mm′ ss.s″:

As the name suggests, in the DMS format latitude and longitude coordinates are denoted with the degrees, minutes and seconds values. Degrees are integer and range from 0° to 90° and 0 to -90° for latitude, and 0° to 180° and 0 to -180° for longitude; minutes are also integer, and seconds can be written as integer or real number (decimal). Note that since 1° = 60′, if you have 60′, you can carry over and add 1° to the degree part instead; and similarly if you have 60″, you can carry over and add 1′ to the minute part instead. Therefore minutes and seconds can always be written less than 60. Example: 45° 36′ 23″ N 122° 46′ 18.4″ W;
46° 73′ 43″ N 118° 36′ 83.6″ W can be written as 47° 13′ 43″ N 118° 37′ 23.6″ W (for latitude coordinate 73′ = 1° 13′ and for longitude coordinate 83.6″ = 1′ 23.6″).

Degrees Decimal Minutes – ddd° mm.mmm′:

Here the degrees are integer values while the minutes are written as real number (decimal). The decimal part holds the fractions of the minute; therefore there is no seconds part. Example: 23° 34.56′ S 109° 23.72′ E

Decimal Degrees – ddd.ddddd°:

In this format latitude and longitude coordinates are denoted only by degrees as real (decimal) number. Any minutes and seconds can be converted to fractions of a degree as decimals. Example: 46.3678° -122.28453° (positive latitude is north, negative longitude is west).

Often times, there is a need for conversion between different geographic coordinate formats. Converting between formats is discussed in detail below. It is helpful to remember some common relations between degrees, minutes and seconds: 60′ = 1°, 30′ = 0.5°, 15′ = 0.25°, and 45′ = 0.75°; similarly 60″ = 1′, 30″ = 0.5′, 15″ = 0.25′, and 45″ = 0.75′.

Convert minutes to degrees: degrees = minutes / 60
Convert degrees to minutes: minutes = degrees x 60
Convert seconds to degrees: degrees = seconds / 3600
Convert degrees to seconds: seconds = degrees x 3600
Convert seconds to minutes: minutes = seconds / 60
Convert minutes to seconds: seconds = minutes x 60

Convert Degrees Minutes Seconds (DMS) to Decimal Degrees

This conversion is accomplished by converting minutes to degrees, as well as converting seconds to degrees, and then adding the results to the original whole degrees. Decimal Degrees = Degrees + (Minutes / 60) + (Seconds / 3600)
Example – convert 38° 12′ 46″ to decimal degrees: 12′ = 0.2° and 46″ = 0.01278°
38° 12′ 46″ = 38° + (12 / 60)° + (46 / 3600)° = 38° + 0.2° + 0.01278° = 38.21278°

Convert Degrees Minutes Seconds to Degrees Decimal Minutes

Seconds need to be converted to minutes, and the result added to the original whole minutes.
Degrees Decimal Minutes = Degrees and (Minutes + (Seconds / 60))
Example – convert 38° 12′ 46″ to decimal degrees: 46″ = 0.767′
38° 12′ 46″ = 38° 12′ + (46 / 60)′ = 38° (12 + 0.767)′ = 38° 12.767′

Convert Decimal Degrees to Degrees Decimal Minutes

In this conversion, the whole number of degrees is known; the decimal part of the degree needs to be converted to minutes. The (-) sign in a decimal degrees coordinate denotes south if the coordinate is a latitude or west if it is longitude.
Example - convert 68.4673° to degrees decimal minutes:
The value of whole degrees is 68°; by subtracting the whole degree from the original coordinate, the fractional part of degrees results (68.4673° – 68° = 0.4673°). Multiply the fractional part of degrees by 60 to convert to minutes.
68.4673° = 68° + 0.4673° = 68° (0.4673 x 60)′ = 68° 28.038′

Convert Decimal Degrees to Degrees Minutes Seconds (DMS)

First the fractional part of degree is converted to decimal minutes as above to get the degrees decimal minutes format. Then the decimal minutes is converted to minutes and seconds. Similar to above step whole minutes and fractional part are separated, and fractional minute is converted to seconds by multiplying it by 60.
Example – convert 68.4673° to degrees minutes seconds:
68.4673° = 68° + 0.4673° = 68° (0.4673 x 60)′ = 68° 28.038′
68° 28.038′ = 68° (28 + 0.038)′ = 68° 28′ (0.038 x 60)″ = 68° 28′ 2″ (seconds rounded off, can be kept as decimal)

Convert Degrees Decimal Minutes to Decimal Degrees

The minute's part is converted to degrees and the result is added to the original whole degrees.
Decimal Degrees = Degrees + (Minutes / 60)
Example – convert 122° 35.483′ to decimal degrees: 35.483′ = 0.59138°
122° 35.483′ = 122° + (35.483 / 60)° = 122° + 0.59138° = 122.59138°

Convert Degrees Decimal Minutes to Degrees Minutes Seconds (DMS)

The decimal minutes part is converted to minutes and seconds. Whole minutes and fractional part are separated, and fractional minute is converted to seconds by multiplying it by 60.
Example – convert 43° 36.847′ to degrees minutes seconds:
43° 36.847′ = 43° (36 + 0.847)′ = 43° 36′ (0.847 x 60)″ = 43° 36′ 50.8″

Latitude Longitude and Datum

A coordinate system may be based on different datums. Different maps may refer to different datums, or the datum referenced by the GPS receiver may not correspond to the one for the map. For example older maps in North America are based on NAD 27 datum while recent maps are based on NAD 83. The default datum for GPS receivers is usually initially set to World Geodetic System of 1984 (WGS 84). And interactive maps such as Google Maps and Bing Maps use the WGS 84 datum.

Example of map datum and coordinate conversion

Transferring coordinates between map versions, GPS and map, or digital and paper maps might cause errors in location of coordinates due to the mismatch of datums. For example transferring coordinates of a trail junction from a map based on NAD 27 datum to a GPS that is set to WGS 84 datum may result in the GPS showing the location of the trail junction couple hundred meters off from the actual location.

It is important to state the datum when working with geographic data. Guide books usually specify the datum for the coordinates they provide. The datum in GPS receivers can be set to correspond to the desired map's datum. The datum is printed on the margin of topographic maps, sometimes along with information for conversion of coordinates between two different datums by addition to or subtraction from latitude and longitude geographic coordinates; such as the example to the right.

Example of change in datum between map versions: http://www.nrcan.gc.ca/earth-sciences/geography-boundary/mapping/topographic-mapping/10129.

Latitude longitude on map – location coordinates

Latitude and longitude (lat long) values are usually marked on the margins of a map. Latitude and longitude lines may also be shown as a grid across the map. Usually only the major divisions are labeled to prevent the cluttering of the map. Sub-divisions may only be labeled with partial coordinate values such as minutes or seconds. There is no one standard way of labeling latitude longitude lines on the map and its margins, and there can be differences depending on the map scale, purpose of the map, and country or locality of production.

Latitude longitude coordinate values on the map margins or a graticule across the map facilitate pinpointing the location of any point given its coordinate values. Similarly geographic coordinates of any point on the map can be derived.

It should be kept in mind that large scale maps may cover areas that span less than a degree of latitude and a degree of longitude. It is helpful to check the four corners of the map to find the coordinates of the bounding area that is covered by the map, especially when latitude longitude values are marked with partial numbers and there is no grid on the map. Also the quadrant or the location with respect to the equator and prime meridian should be known when reading values on the map margin. For example in an area in northern and western hemisphere such as USA or Canada, the latitude values increase from 0° at equator to 90° at north pole while longitude values go from 0 to -180° (the absolute number increases) traveling west. In southern and eastern hemisphere, latitude values go from 0° at equator to -90° (the absolute number increases) at south pole, and longitude values increase from 0° at prime meridian to 180° at International Date Line.

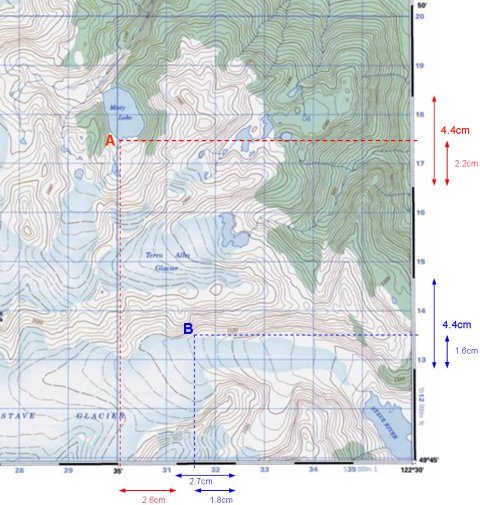
The map used in this example is a 1:50000 topographic map of a region in British Columbia, Canada. The map sheet covers an area from 49°45′ N to 50°00′ N latitude and from 122°30′ W to 123° W longitude; the image belongs to the lower right hand corner of the map. In this map, the blue gridlines on the map and the blue consecutive numbers labeling each grid line are the UTM coordinates. Looking at the margins and the black numbers denoting geographic coordinates, it is evident that not every degree or minute is labeled; therefore it is important to examine the labels to find out the length of the divisions. In this map each of the alternating black and white bars on the margin represents 1′ of latitude running north–south and 1′ of longitude running east–west.

Keep in mind that the grid lines or known margin divisions of latitude and longitude may be different in different maps. For example latitudes may be divided into 1′ sections and longitudes into 2′ intervals.

Find latitude longitude coordinates of a location on map

© Natural Resources Canada

Based on the definition of latitude and longitude, to find the geographic coordinates of a point on a map, the angular distance (north – south) of the point relative to the equator and its angular distance (east – west) relative to the prime meridian should be determined.

In this example the geographic coordinates of the point of inflow of a stream into Misty Lake from the south, designated as point A is desired. The first step is to find out the nearest latitude and longitude whole values around point A from the gridlines or in this case from the margins of the map. The latitude longitude values of point A on the margin can be marked by placing a straight edge from point A perpendicular to the margins. From the right margin it is clear that point A is between 49°48′ N and 49°49′ N latitude, and the bottom margin shows the longitude of point A to be between 122°34′ W and 122°35′ W.

The latitude and longitude values of point A can be easily estimated by eyeballing their relative position compared with the nearest surrounding known latitude longitude values. Each bar can be visually divided into ten equal sections. Here point A's latitude is halfway between 49°48′ N and 49°49′ N latitude. In other words, its minute section is about 0.5′ greater than 48′. Adding this angular distance to the lower latitude, point A's latitude would be 49°48.5′ N. Similarly point A's longitude can be estimated to be about 0.9 minutes greater than 122°34′ W, which can be stated as 122°34.9′ W.

A ruler can be used in order to obtain more precise coordinate values for the point. Measuring the distance between the point marking the latitude of point A to the immediate known lower latitude value, and dividing this distance by the distance of the section between lower and higher latitude values result in the proportional distance of point A from the known lower value. This distance is added to the lower latitude to give the latitude of point A.

In this example using a ruler, point A's latitude distance from the known lower latitude (49°48′ N) is 2.2cm. The distance between the lower (49°48′ N) latitude and higher (49°49′ N) latitude is 4.4cm. Here 1′ of latitude = 4.4cm. The proportional distance of A's latitude to lower known latitude is 2.2 / 4.4 = 0.5. Converting this value to angular distance (in this case angular distance between the lower and higher latitude is 1′ = 60″): 0.5 x 1′ = 0.5′ or 0.5 x 60″ = 30″. Adding this value to the lower latitude provides the latitude of point A: 49°48.5′ N or 49°48′ 30″ N. Point A's longitude is calculated in the same fashion. Distance of A's longitude from known lower longitude (122°34′ W) is 2.6cm. The length of the section between lower (122°34′ W) and higher (122°35′ W) longitudes is 2.7cm. Here 1′ of longitude = 2.7cm. Proportional distance is 2.6 / 2.7 = 0.963cm. Converting this distance to angular distance: 0.963 x 1′ = 0.963′ or 0.963 x 60″ = 57.78″. Adding this value to the lower longitude provides the longitude of point A: 122°34.963′ W or 122°34′ 57.8″ W. Point A's geographic coordinate: 49°48′ 30″ N 122°34′ 57.8″ W.

Plot a point with known geographic coordinates

In order to locate a point on a map given its geographic coordinates, its latitude and longitude values need to be marked on the margins or gridlines on map. The point can then be placed at the cross section of straight lines perpendicular to the margin originating from marked latitude longitudes. In this example coordinates of point B are known: 49°46′21.8″ N 122°33′40″ W.

These values can be marked by eyeballing their relative position compared with the nearest surrounding known latitude longitude values. 21.8″ = 0.363′, therefore latitude of B can be written as 49°46.363′ N. Visually dividing the bar into ten sections (each would be 0.1′), and estimating the distance of 0.363′ or 0.4′ on the bar, it is easy to locate the position of the latitude on the margin. Similarly 40″ = 0.667′, so the longitude is 122°33.667′ W. And 0.667 or 0.7 can be estimated on the bar after 122°33′ mark.

A ruler can be used in order to pinpoint these values on the margins. 21.8″ = 0.363′, this fraction of a minute can be converted to proportional distance in cm of a bar. A bar is 1′ and equal to 4.4cm, therefore the proportional distance is 0.363 x 4.4 = 1.597cm. The latitude of B can be marked about 1.6cm to the north of 49°46′ N point. Similarly for longitude, 40″ = 0.667′, and 0.667 x 2.7 = 1.8cm, therefore the longitude of B can be marked around 1.8cm to the west of 122°33′ W point. Perpendicular lines to the edges of the map drawn from these points cross at point B.